Jewel
Contributed by Basudeo Agarwal
This is a ‘draw and discard’ game and has some resemblance to Indian Rummy. Monetary stakes can be added to the game to make it more charming and thrilling.
PLAYERS:- The "full board" is of nine players, though the game can be played by only two players. Therefore, any number of players from two to nine can play this game. Before start of the play, it is necessary to ‘cut for seat’. That is, every player picks a single card from the deck. The player pulling the highest card chooses his seat at the table and the rest follow him clockwise in descending order. The puller of the highest card deals first. If the board is full, that is nine players are playing, there should be a new cut for seat after every round of nine deals. Otherwise, the house should determine in advance the minimum number of rounds necessary before ‘cut for seat’ must be repeated.
DECK:- Three simple playing card decks are necessary for this game. A deck must have usual 52 cards and two printed JOKERS. So a deck will have, 52+2 = 54 cards. So three decks will have 54x3 = 162 cards in total.
DEAL:- After shuffling the cards thoroughly, the dealer offers the deck to the player to his left to cut. After the ‘cut’ the dealer puts the cut cards below the uncut cards and offers the deck, face down, half spread on the table, to the player to his right for the drawing of the ‘Open Card’. The player takes a single card from the middle of the deck and puts that card face up in the centre of the table.
- OPEN CARD :- A joker can never be an open card. So every time a joker is drawn, it is inserted back in the deck and a fresh card is pulled. (More about ‘open card’ in ‘definitions’.)
After the successful drawing of the open card, the dealer distributes the cards face down one at a time in a clockwise direction. The first card is dealt to the player to his left and the last card to himself. 16 such rounds are dealt, that is 16 cards to every player. After distribution of the cards, the dealer stacks the remaining cards face down on top of the ‘open card’ not concealing the ‘open card’ fully. This face down stack is known as the ‘stock’. No ‘Chance Card’ is exposed in this game to start the discard pile. as in some other variations of rummy type games. The discard pile begins empty. The turn to deal passes clockwise.
DEFINITIONS:- First let me give definitions of the terms used in this game. These definitions are in context of this game "JEWEL" only.
- JOKER:- there are six printed jokers, two from each deck. Jokers can be used as substitutes for any card but with some limitations, discussed later.
- OPEN CARD:- A card pulled by the player to the right of the dealer at the start of the deal. A joker can never be an open card. The ‘open card’ determines the JEWELS and the construction of the ‘Super Sequence’ and ‘Marriage’, as defined below.
- JEWEL:- one of the six cards of the same rank but of the opposite color to the ‘open card’. For example if
 7 is the ‘open card’, then the three
7 is the ‘open card’, then the three 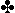 7’s and the three
7’s and the three  7’s are JEWELS. A jewel can also be used like a joker, as a substitute for any card, but with the same limitations that apply to jokers. JEWELS also allow the holder to score bonus points, as discussed in Points. The holder is free to use a jewel or jewels as jokers or as original cards. For example:
7’s are JEWELS. A jewel can also be used like a joker, as a substitute for any card, but with the same limitations that apply to jokers. JEWELS also allow the holder to score bonus points, as discussed in Points. The holder is free to use a jewel or jewels as jokers or as original cards. For example:  7 being the open card,
7 being the open card, 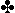 4 -
4 - 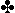 5 -
5 - 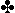 6 -
6 - 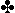 7 is considered a four card pure
sequence and the holder will also get bonus points for
7 is considered a four card pure
sequence and the holder will also get bonus points for 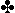 7, the jewel. Similarly
7, the jewel. Similarly 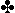 7 -
7 - 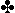 7 -
7 - 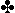 7 is a 'super set' and the holder will get bonuses for both 'jewels' and 'super set'.
7 is a 'super set' and the holder will get bonuses for both 'jewels' and 'super set'. - SPOT CARDS:- All the cards from the two spot to 10 spot are called spot cards. Running order is 2 - 3 - 4 - 5 - 6 - 7 - 8 - 9 - 10.
- HONOR CARDS:- All the cards from jack to ace are called honor cards. Running order is jack - queen - king - ace.
- SEQUENCE:- A sequence is of three cards in running order of the same suit. In this game JEWEL, a sequence must consist either of spot cards or of honor cards. It cannot be a mixture of the two groups. Valid sequences : 2 - 3 - 4, 5 - 6 - 7, 8 - 9 - 10, J - Q - K etc. Invalid sequences : 9 - 10 - J, 10 - J - Q etc. Joker/jewel can be used.
- COMPULSORY SEQUENCE:- A pure sequence (without the aid of any jokers or jewels) of four cards from either group is a ‘compulsory sequence’. As the name suggests, players are obliged to hold such a sequence, otherwise their full hand will be counted for penalties. There is only one possible compulsory sequence from the honor card group: J - Q - K - A.
- AUXILIARY SEQUENCE:- This is only necessary for making a declaration. It must be of three cards only from either group and without the aid of any joker or jewel. For a defender (a player other than the one who declares) it is not necessary to have an auxillary sequence to avoid the full hand penalty.
- SUPER SEQUENCE:- A super sequence consists of exactly three cards, with the middle card identical to the 'Open Card’. Thus two ‘super sequences’ are possible. In the given example as diamond 7 is our open card; two sequences of diamond 6 - 7 - 8 are possible, since one of the three diamond 7's in the deck is already under the stock, showing the ‘open card’. The restriction on being from one group is not applicable in case of a super sequence. Suppose the diamond 10 is the ‘open card’: then diamond 9 - 10 - J is a super sequence. A ‘Super Sequence’ scores bonus points and these bonus points are only allowed when the super sequence consists of exactly three cards without the aid of any joker or jewel. It also serves the purpose of ‘auxiliary sequence’ for declaration if and only if it is entirely from one group. In the first example above, if holding the diamond 6 - 7 - 8, the declarer does not require any further ‘auxiliary sequence’ and he will get the bonus points for a super sequence. However, in case of diamond 9 - 10 - J, his three cards are arranged and he will also get the bonus for a super sequence, but it will not serve the purpose of an ‘auxiliary sequence’. In the absence of a separate ‘compulsory sequence’, one can make a super sequence serve the function of a compulsory sequence also by adding one more card to it. However, in this case the whole sequence must be from one or the other group, and by doing so one will forfeit the bonus points associated with the ‘super sequence’. Please note: if the open card is
- A 2 spot - the ‘super sequence' is A - 2 - 3.
- An ace - the ‘super sequence' is K - A - 2.
- SET:- Three cards of the same rank but of different suits are known as a ‘set’. Jokers or jewels can be used as substitutes.
- SUPER SET:- Three cards of same rank and of the same suit make a ‘super set’. In a super set, jokers and jewels cannot be used. A super set also serves the purpose of ‘auxiliary sequence’ for the declaration. A "Super set" also scores bonus points as discussed in ‘points’.
- MARRIAGE:- A Marriage is the combination of the king and queen in the other suit of the same color as of ‘open card’. For example if the open card is from the diamond suit then a ‘marriage’ is only possible in the heart suit and vice versa. Similarly in black suits. In the example above, where the
 7 is the open card, the hearts King and Queen make a ‘marriage’. Thus, three marriages in total are possible, since there are three copies of each card. Bonus points are associated with ‘marriages’. To earn the bonus points the King and Queen must be in combination - i.e. bonus points are allowed only when both cards are used in the same sequence. A joker or jewel may be used to complete the sequence but the original king and queen must be there to earn bonus points. The king and the queen used in different ‘sets’ do not count for bonus points, though those are valid arrangements of the cards. If J - Q - K or Q - K - A is pure, it will also serve the purpose of auxiliary sequence for declaration and will earn bonus points. Similarly, if J - Q - K - A is pure it will serve the purpose of compulsory sequence and will earn bonus points.
7 is the open card, the hearts King and Queen make a ‘marriage’. Thus, three marriages in total are possible, since there are three copies of each card. Bonus points are associated with ‘marriages’. To earn the bonus points the King and Queen must be in combination - i.e. bonus points are allowed only when both cards are used in the same sequence. A joker or jewel may be used to complete the sequence but the original king and queen must be there to earn bonus points. The king and the queen used in different ‘sets’ do not count for bonus points, though those are valid arrangements of the cards. If J - Q - K or Q - K - A is pure, it will also serve the purpose of auxiliary sequence for declaration and will earn bonus points. Similarly, if J - Q - K - A is pure it will serve the purpose of compulsory sequence and will earn bonus points.
RULES:- This is a draw and discard type game: a turn consists of drawing one card and discarding one card face up on the discard pile. The objective of every player is to arrange their hand of 16 cards in a set pattern. As soon as the deal is over, the player sitting left to the dealer gets first opportunity to play. The discard pile is empty at this point, so he does not get any chance to take a known face up card. If he thinks that he will be able to collect a hand that can be sufficiently well arranged into sets and sequences to score points, he can play by drawing a single card from the ‘stock’. Alternatively he can go ‘pack’ (retire from the play). If the first player plays then from that poiint onwards everybody will have the option either to draw an unknown card from the ‘stock’ or pick the known card discarded by the previous player from the discard pile. If the first player packs, then the second player's only option, if he wishes to play, is to draw from the stock, and so on.
If a joker or jewel is discarded (knowingly or unknowingly) it cannot be picked up by the next player.
- ARRANGEMENT OF CARDS:- The pattern for arrangement of 16 cards is exactly :- 4 + 3 + 3 + 3 + 3 and nothing else. The 4-carder must be a pure sequence from either group. In addition, the rest of the hand must consist of exactly 4 groups of three cards only. These 4 groups may be sequences, sets, or both. The arrangement of cards necessary for declaration will be further clarified in Declaration.
- PACK:- This is a term used for not playing a particular hand. A player must decide at his first opportunity either to play or to go pack. If a player wants to go pack he should insert his cards face down at the bottom of the ‘stock’, but if he holds any ‘jewels’ he may keep one or two (but not more) of them face up in front of him on the table, as they score bonus points. Since every player must get a chance to go pack, no declaration can be made in the very first round of play, except that the dealer can make this declaration at his first opportunity, since every other player has had the chance to go pack before the dealer's first turn to play. A player who wishes to go pack cannot draw any card from the ‘stock’ or from the ‘discard pile’.
- MID PACK:- In this game JEWEL there is also the opportunity to go pack in the middle of the game, but with some restrictions. If the board is full - that is 9 players are playing - then ‘mid pack’ is allowed only after the completion of at least two rounds of play. For this purpose, a round starts from the player who wants to go ‘mid pack’ and ends at the player to his right. If there are fewer than noine players, then the house should decide in advance the minimum number of rounds before a ‘mid pack’ is allowed. Mid pack is allowed only on the turn of that player. On the turn, he only sees the known card in the discard pile but in no case can he see the unknown card from the stockpile. If a player wants to go mid pack he should insert his cards face down at the bottom of the ‘stock’ after keeping a maximum ‘jewels’ from his hand face up in front of him on the table.
- DECLARATION:- When a player arranges all 16 cards in set order, he can make declaration. However, he must make the declaration on his turn only after drawing a card either from the stock or from the discard pile. He makes the declaration by thumping his discard face down on the discard pile. He must make sure that his hand satisfies these requirements:-
- A 4 carder compulsory pure sequence from either group.
- An auxiliary pure sequence of three cards from either group. THis may be a ‘super sequence’ if it is from one group, or a ‘super set’.
- The pattern of arrangement of his 16 cards is exactly - 4 + 3 + 3 + 3 + 3.
- STOCK:- A fresh 'stock' is created if the 'stock' is exhausted. The player who draws the last card from the 'stock' creats the fresh 'stock'. He leaves his discard face up in the discard pile and collects all other cards from the discard pile. He shuffles them well and offers these cards for 'cut' to the player sitting at his right (who may be a 'pack' player). He puts cut cards below uncut cards, and this is the fresh 'stock'. The game must be completed before this second 'stock' also exhausts. Otherwise, the hand is treated finished 'without declaration'. All 'pack' and 'mid pack' players get releif from paying the pack value to the declarer as there is no declarer. Playing hands count their points for unarranged cards and settle the account on a difference basis. Jewels and bonuses for playing hands are rewarded as usual.
POINTS:- This is a point-based game so utmost care should be taken on various points.
[Editor's note - the 'difference based' scoring system used in this game is rather complex. I found it easiest to understand by thinking of all the scores as payments between players. Sometimes an amount is paid to one opponent, sometimes to several and sometimes to all. My comments in italics and square brackets are an attempt to clarify the explanation of the scoring in these terms. JMM]
- BONUS POINTS FOR ALL HANDS:- The name of the game is JEWEL and there are only 6 jewels. Jewels carry bonus points in the following manner:-
- 2 bonus points for a single jewel.
- 5 bonus points for the possession of two jewels of the same suit.
- 10 bonus points for the possession of all three jewels of the same suit.
[Points for jewels are paid to the holder of the jewel by everyone at the table. JMM] - BONUS POINTS FOR PLAYING HANDS:- These bonus points are only for those who play the hand till the end. Pack or mid pack hands will neither get these nor do have they to pay for these.
- SUPER SEQUENCE:- 4 points for a super sequence. A player may have both super sequences but he will not get any additional benefit for that and will get just 8 points. As stated previously, a super sequence must be of exactly three cards, the middle card of the sequence being identical to the open card, and without the aid of any joker or jewel. To get the bonus for a super sequence, it is not necessary to have a ‘compulsory sequence’.
- SUPER SET:- 2 points for each super set. A super set of jewels also scores this bonus in addition to 10 bonus points for holding three jewels of the same suit but at the sacrifice of three jokers. A player may have any number of super sets in his hand but without any kind of additional benefit. To get the bonus for a super set, a ‘compulsory sequence’ is not necessary.
- MARRIAGE:- 3 points for each marriage. To get the bonus for a marriage or marriages, you must have a ‘compulsory sequence’.
- PENALTY POINTS:- This game is played on the ‘difference basis’. I will make it clear in example at the end.
- INITIAL PACK:- 4 penalty points for initial pack. Only declarer will get these points. These points are not for any other playing hand on difference basis.
- MID PACK:- 8 penalty points for mid pack are only for the declarer, not for any other playing hand on difference basis.
- FULL HAND:- 12 points for full hand irrespective of total count. These are only for the playing hands and on difference basis. Conditions for full hand
- Having no compulsory sequence.
- Declarer making wrong declaration. Therefore, it is not wise to expose your hand immediately as soon as declaration is made by a player. First it should be thoroughly examined by the rest of the players. A player making a wrong declaration forfeits the right to play any further in that particular hand. The discard card thumped face down by the player becomes chance card for the next player and play continues till any other player makes valid declaration. The hand of the player, who has made the wrong declaration is treated like a hand having no compulsory sequence. He will get bonuses for jewels, super sequence and super set if any, but no bonus for marriage.
- COUNTING POINTS:- Counting of points is done only for hands having a compulsory sequence. The basis of counting is as follows:
- 1 point for every honor card.
- 0.5 point for every spot card. As the final count must be a whole number, the odd 0.5 for an odd number of cards is ignored. For example 5 spot cards count only 2 points.
- Any Jewel or joker is a free card, counting zero. In any case, there is only a very remote possibility that a jewel or joker remains idle in a hand having a compulsory sequence. Counting points are liable for penalty on difference basis.
[A player who packs pays a penalty only to the player who ends the play by making a declaration. JMM]
EXAMPLE HAND:- 9 payers A, B, C, D, E, F, G, H, I are playing JEWEL. In the ‘cut for seat’, A picked the highest card and chose his seat at the table. B picked next highest and so on. ‘A’ had the right to deal so he offered deck to ‘B’ for cut. After ‘cut’, he offered deck to ‘I’ for pulling the ‘open card’. Let us pull the highest card, the ‘ace of spades’ as the open card. The deal is completed by ‘A’ distributing 16 cards to each player.
‘B’ had the first turn to play. He drew a card from the stock and discarded a card. ‘C’ also drew a card from stock and discarded one. ‘D’ picked the card discarded by ‘C’. ‘E’ and ‘F’ went pack, only ‘F’ exposing 1 heart ace in front of him. ‘G’, ‘H’, ‘I’ and ‘A’ played. In his third turn ‘G’ went mid pack and in 4th turn ‘H’ went mid pack, exposing 1 diamond ace in front of him. Then play continued until ‘A’ made a declaration by putting his discarded card face down. Everyone checked and his declaration was all right.
A:- Declaration with:-
- Spade K - A - 2 = super sequence. = 4 bonus for B,C,D, I
- Club Q - K - Joker = marriage = 3 bonus for B,C,D, I
( + 7 hand for playing hands BCDI and nil for EFGH)
[In other words, A is paid 7 points for his bonuese by the other players who stayed to the end, but not by those who packed. JMM]
B:- He had a compulsory sequence and two sets of 3 spots and 6 spots and a set of 9 spots with a joker. He also had club Q - K - Jewel (diamond ace). See, all 16 cards were arranged but he could not make a declaration on his turn because he did not have an ‘auxiliary sequence’. He had a single chance to get an auxiliary sequence either by drawing either a club jack or by drawing a club ace. However, for playing hands, it made no difference but he lost 2x4 + 2x8 = 24 points of packers. His hand :- (+ 5 for ACDI and + 2 for EFGH.)
- Club Q - K - Jewel = marriage = 3 bonus for ACDI.
- Jewel (1 diamond ace) = 2 bonus for every body else.
[B wins 2 points from each other player for his jewel and an extra 3 points from those who did not pack for his bonuses. The slightly cryptic comment 'lost 24 points of packers' refers to the fact that if he had been able to declare before A, the 24 points' worth of packer penalties would have been paid to B rather than to A. JMM]
C:- Unlucky fellow does not have a ‘compulsory sequence’. However, he does have club Q - K - A, but will not get any bonus points for the marriage as he does not have a ‘compulsory sequence’. However, this fellow is lucky to have 2 heart aces and 1 diamond ace.
- + 7 hand for packers, that is for EFGH.(5 + 2)
- - 5 hand for ABDI (12 - 7 = 5)
[7 is the total value of C's jewels. He wins this amount from everyone but also has to pay 12 - the 'full hand' penalty to those who did not pack, making a net payment of 5 to each of them. JMM]
D:- This fellow has a ‘compulsory sequence’: spade J - Q - K - A. He also has a 'super set' of three twos of diamonds, but nothing else. However, he also has a spade 2 but he chooses to show his ‘compulsory sequence’ rather than his ‘super sequence’ He has a total 9 unarranged cards - all 9 are spot cards. His total point count is 4 and he will get 2 bonus points for a ‘super set’.
- Nil hand for packers EFGH.
- - 2 hand for ABCI (4 - 2 = 2).
[He gets 2 bonus for his compulsory sequence but has to pay 4 for unmatched cards. This payment goes only to the players who did not pack. Note that if he had shown his super sequence instead, he would have had to pay the other four surviving players 6 each instead of 2 - winning 6 in bonuses but paying a full hand penalty of 12. JMM]
E:- ‘E’ packed initially without declaring any ‘jewel’. So his hand is -4 for A and nil for every body else.
[Since A declared, E has to pay 4 points to A for packing. JMM]
F:- ‘F’ also packed initially but declared one ‘jewel’ (heart ace). So his hand is - 2 for A and + 2 for every body else.
[Everyone pays F 2 points for the jewel but F pays A 4 for packing. JMM]
G:- ‘G’ went mid pack at his first opportunity to do so. As two rounds were compulsory to play and he went pack on his 3rd turn without any ‘jewel’. So his hand is - 8 for A and nil for every body else.
H:- ‘H’ also went mid pack but declaring 1 ‘jewel, that is the diamond ace. He also took one more chance but surrendered on his 4th turn. His hand is - 6 for A and + 2 for every body else.
I:- This fellow was dealt two ‘super sets’ of spade 5 and club 7. He hung onto them until the end but finished without a ‘compulsory sequence’.
- Nil hand for EFGH.
- - 8 hand for ABCD (12 - 4).
That is, I must pay 12 for 'full hand' to the playing hands but also claims 4 from the playing hands. So net - 8 for playing hands.
Here is the summary of all the hands:
| PLAYER | JEWELS | BONUS | PENALTY |
|---|---|---|---|
| A (Dec.) | NIL | 7 | NIL |
| B | 2 | 3 | NIL |
| C | 7 | NIL | - 12 |
| D | NIL | 2 | - 4 |
| E (Pack.) | NIL | (4) | |
| F (Pack.) | 2 | (4) | |
| G (M.Pack) | NIL | (8) | |
| H (M.Pack) | 2 | (8) | |
| I | NIL | 4 | -12 |
| TOTAL: | 13 | 16 | (24) - 28 |
I am giving all such details to make the understanding of the game easy. But settling of the account at the finish of a hand is very easy. People generally play with tokens. A supply of tokens worth 1 point, 2 points , 5 points and 10 points, and see how easily the account is settled.
As 'A' is the declarer, he collects (7 - 5 ) 2 points from "B', (7 + 5) = 12 from "C" etc.
Then "B" starts collecting from "C" (5 + 5) = 10 etc.
Then "C", then "D" etc.
It will take hardly 1 or 2 minutes.
Some people may like to play the game without money or tokens and want to play with paper and pen; account to be settled at the end of the session, at some latter date or never. It will require little computation. Points to remember:-
- Have a total count of points for jewels. In the example all 6 jewels are dealt, with one pair. So there are 13 total points for 'jewels'. So each of the 9 players must make a payment of 13 points. Wait ! Take the example of "F". He packed with a jewel so +2 to his credit. He collects 8 x 2 = 16 and pays 11 points for the jewels held by other players. So his net profit for jewels is 5 (16 - 11). But it can also be calcuted in the following manner:- He gets 9 x 2 = 18 and pays 13 points for total jewels. The result is the same. You will see, it is more logical.
- Have a total count of bonus points for playing hands. In the example: 1 super sequence, 2 marriages and 3 super sets giving total count of 16 points. So each of playing hands must pay 16 points.
- Have a total count of penalty points. In the example hand it is: C=12, D=4 and I=12. That is total 28 points. So each of playing hand will get 28 points.
- As the amounts in (2) and (3) are only paid to the playing hands, we calculate the 'net'. Net for playing is hands +16 (bonus) - 28 (penalty) = - 12. So each of playing hand will get these 12 points considering penalties and bonuses for playing hands.
- Again (1) is meant for all players. So we take the 'net' of (1) and (4). Net of 'jewels' and (4) = 13 - 12 = 1. So each playing hand has to pay 1 point.
Now the calculation:-
There are 9 players in total and 5 playing hands.
-
A's share 7x5 - 1 = 34 and 4+4+8+8 of pack going players gives 34+24= 58
B's share 2x9 + 3x5 - 1 = 32
C's share 7x9 - 12x5 - 1 = 2
D's share - 2x5 - 1 = - 11
E's share - 13 - 4 = - 17
F's share 2x9 - 13 - 4 = 1
G's share - 13 - 8 = - 21
H's share 2x9 - 13 - 8 = - 3
I's share - 8 x 5 - 1 = - 41
Check: (58 + 32 + 2 + 1) = 93 and (11 + 17 + 21 + 3 + 41) = 93 . Gains and losses are equal, as expected.
Here is a chart showing the account settlement in another way.
| Player | Score | A | B | C | D | E | F | G | H | I | TOTAL |
|---|---|---|---|---|---|---|---|---|---|---|---|
| A | (0 + 7) | X | 2 | 12 | 9 | 4 | 2 | 8 | 6 | 15 | 58 |
| B | 2 + 3 | - 2 | X | 10 | 7 | 2 | 0 | 2 | 0 | 13 | 32 |
| C | 7 - 12 | -12 | - 10 | X | - 3 | 7 | 5 | 7 | 5 | 3 | 2 |
| D | 0 - 2 | -9 | - 7 | 3 | X | 0 | - 2 | 0 | - 2 | 6 | - 11 |
| E | 0 (4) | - 4 | - 2 | - 7 | 0 | X | - 2 | 0 | - 2 | 0 | - 17 |
| F | 2 (4) | - 2 | 0 | - 5 | 2 | 2 | X | 2 | 0 | 2 | 1 |
| G | 0 (8) | - 8 | - 2 | - 7 | 0 | 0 | - 2 | X | - 2 | 0 | - 21 |
| H | 2 (8) | - 6 | 0 | - 5 | 2 | 2 | 0 | 2 | X | 2 | - 3 |
| I | 0 - 8 | - 15 | - 13 | - 3 | - 6 | 0 | - 2 | 0 | - 2 | X | - 41 |
[The score column for the players who did not pack shows two numbers. The first represents points that they win (for jewels) from every other player; the second represents the additional amount they win from players who did not pack, for bonuses less penalties. A negative number here represents a payment to each other player. A's scores are in brackets, perhaps to indicate that he is the player who declared.
The players who packed also have two numbers in the score column. The first is the amount they win from everyone for jewels; the second, in brackets, is the amount they pay to the player who declared for packing. This bracketed number is really negative for the player in whose row it appears.
In the body of the table, the net payment between each pair of players is calculated. For example D has a score of -3 so must pay 3 to B; B has a score of +5 so collects an additional 5 from D. Therefore the number 8 appears in B's row and D's column representing the total of 8 that B gets from D, and the balancing figure of -8 in D's row and B's column represents the same payment from the point of view of D, who has to pay it.
The totals on the right show the toal amount won or lost by each player on the deal. These numbers will always balance, with a total of zero. JMM]